Previous
Topic

|
Exploring whole numbers ( Number Operations & Number Theory )
Term :
1
Module : 1 - Capturing Data
Unit 1
: Numbers Made Easy
( Rational Numbers ) -
Properties &
Special Operations
CONVERTING
FRACTIONS
|
|
Mixed numbers
To
Improper fractions
|
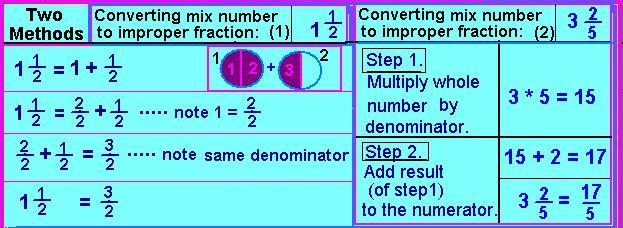
When working with fractions, we sometimes need to convert mixed numbers to
improper fractions.
(We should note that a whole number is the same as that same number over
1. (eg. 3 = 3/1) )
In the examples below, two methods are used :
In
Method 1,
the whole part of the mixed number is expressed as a fraction.
This fraction is then added to the fraction part of the mixed number.
In
Method 2,
the whole part of the mixed number is multiplied by the denominator of the
part of the mixed number that is a fraction. This result is then added to
the numerator, and placed over the denominator of the fraction. |
|
|
Examples
|
 |
|
Improper fractions
To
Mixed numbers
|
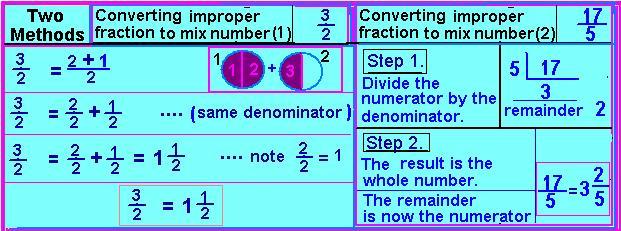
When working with fractions, we sometimes need to convert improper fractions
to mixed numbers.
(We should note that a whole number is the same as that same number over
1. (eg. 3 = 3/1) )
In the examples below, two methods are used :
In
Method 1,
the numerator is broken up into 2 numbers. One of these numbers is the
largest whole number less than the numerator, that the denominator can
divide evenly. The other number is the resulting remainder of this division.
Both these numbers are placed over the denominator giving the parts of the
mixed number that is the whole number, and the fraction.
In
Method 2,
the numerator of the improper fraction is divided by the denominator .
The Quotient becomes the whole number part of the mix number.
The Remainder now becomes the numerator of the fraction part of the mix
number, and the denominator remains the same. |
|
|
Examples
|
 |
|
Fractions
To
Decimals
|
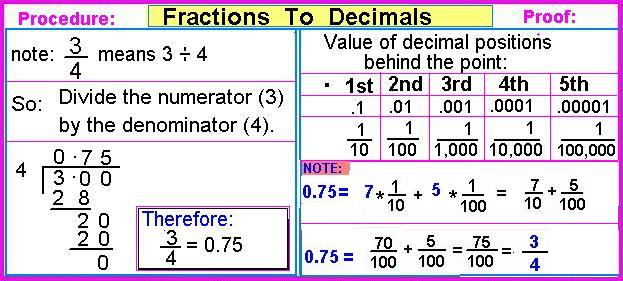
When working with fractions, we sometimes need to convert fractions to
decimals.
(We should note that a whole number has an implied decimal point to the
right. (eg. 3 = 3. ) )
This method simply involve dividing the numerator by the denominator.
In performing this division, we must place a decimal point after the number
and add zeros as necessary since this does not change the value of the
original number.
Continue the division until there is no remainder, or until you get a
repeating remainder.
The result of this division is the required decimal number.
(note the fraction 3/4 is really 3 divided by 4). |
|
Example
|
 |
|
Decimals
To
Fractions
|
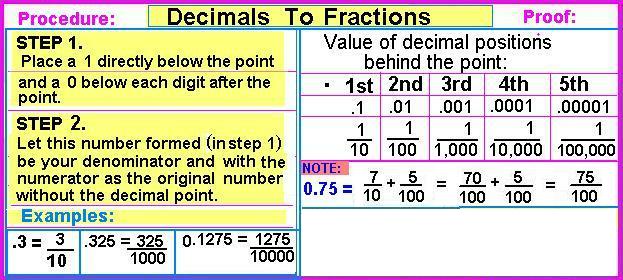
When working with fractions, we sometimes need to convert decimals to
fractions .
This method involve 2 simple steps as seen in the example below.
(i) Treat the decimal number as the numerator of the fraction.
(ii) Form the denominator of the fraction by placing a 1 under the decimal
point, and a zero under each digit of the numerator. (now remove the decimal
point from the numerator).
|
|
Example
|
 |
|

Back To Quiz |
TOPICS
 |
|